How to Find Average Velocity Physics

It is essential to comprehend velocity in both science and daily life. It aids in describing the direction and speed of an object’s motion. In instance, average velocity sheds light on an object’s total motion throughout a specific period of time. We will examine the idea of average velocity, its importance, and step-by-step procedures to precisely calculate it in this comprehensive tutorial.

What Is Average Velocity

A measurement of an object’s displacement over a certain period of time is its average velocity. Both the magnitude (speed) and direction of motion are taken into account. Velocity is a vector quantity, meaning it has both magnitude and direction, as opposed to speed, which is a scalar number.

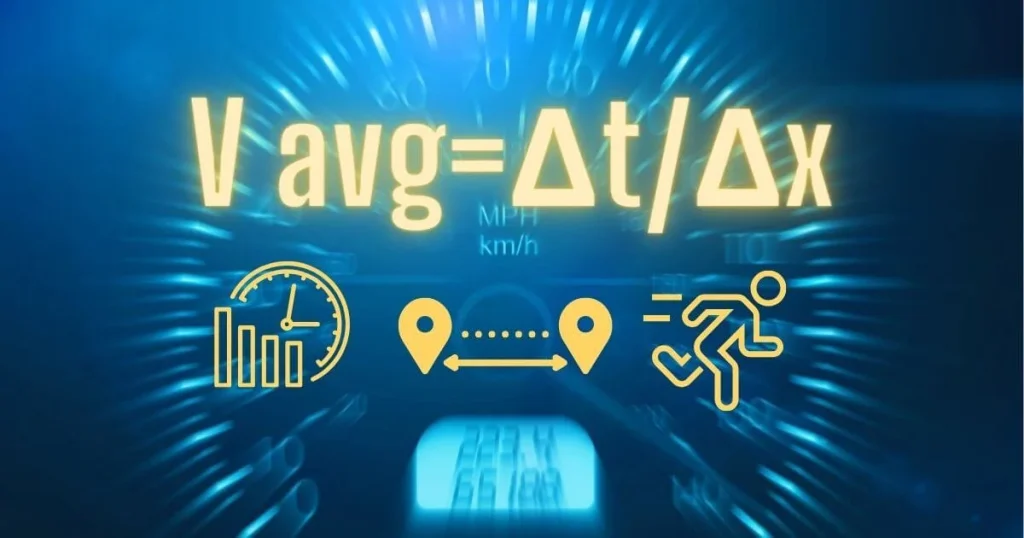
Average velocity (V_avg) is computed mathematically using the following formula:

Average Velocity Formula

vav  = Δx/Δt= V average = t x

Where:

The average velocity is shown by the letter vav

The object’s change in displacement or location is represented by the symbol Δx

It is the shift in time that causes the displacement Δt

Why Average Velocity Matters:

• Physics and Kinematics:

A key idea in physics is average velocity, especially in the field of kinematics, which examines the motion of objects. It enables systematic analysis and description of object motion.

• Real-World Applications:

Understanding average velocity is crucial in a variety of real-world situations, like figuring out a car’s speed during a road trip, an athlete’s average speed during a competition, or an airplane’s velocity during a flight.

• Problem Solving:

In order to solve issues involving motion, displacement, and time, average velocity is a necessary tool. It aids in our ability to respond to queries like “How far did the object travel?” the question “What was its overall speed?”

How to Calculate Average Velocity formula : 

Step-by-Step Guide

Let’s now examine the procedure for calculating average velocity step by step:

Step 1: Gather Data

Two pieces of data are required to compute average velocity:

• Initial Position (xi):

Usually measured in meters (m) or another unit of length, this is where the object’s motion begins.

• Final Position (xf):

The object’s final location, expressed in the same units as its starting position, is at this point in its motion.

• Time Interval (t):

Find the period of time when the motion took place.This is the difference between the ending time (tf)

and the starting time (ti​).

Step 2: Calculate Displacement (Δx)

The displacement (Δx) is the change in position or the difference between the final position and the initial position: 

Δx=xf​−xi

​Step 3: Calculate Average Velocity (Vavg)

Use the formula for average velocity:

vav  = Δx/Δt

Step 4: Include Direction

Velocity has magnitude and direction since it is a vector quantity. When expressing average velocity, be sure to specify the direction. Depending on the context of the motion, you can use positive and negative signs or specific directions like “east,” “north,” etc.

Example: how to Calculating Average Velocity

Let’s go over an example to see how average velocity is calculated:

Scenario: A car travels from point A to point B, covering a distance of 100 kilometers in 2 hours.

Step 1: Gather Data

• Initial Position  (xi) Point A
• Final Position (xf) Point B
• Time Interval (t) 2 hours

Step 2: Calculate Displacement

Δx=xf−xi = 100km − 0km =100km

Step 3: Calculate Average Velocity

Vavg  = Δx / Δt  = 100km / 2h = 50km/h

Step 4: Include Direction and Average Speed

In this case, the car is moving from point A to point B, so the average velocity is 50 km/h in the direction from A to B.

Conclusion:

A fundamental idea in physics, average velocity is a key term for defining how things move. We may learn more about an object’s overall motion, including its speed and direction, by computing average velocity. Finding average velocity is a useful ability that enables us to make sense of the dynamic world around us, whether we’re studying the motion of an automobile, an athlete, or any other moving item.

visit: average velocity calculator